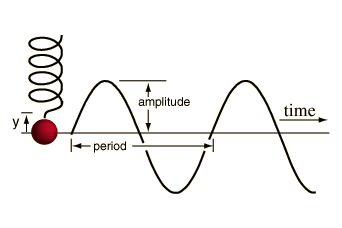
An example of such an oscillatory motion is Simple Harmonic Motion. Simple harmonic motion describe the neatest form of.
Any system that obeys simple harmonic motion is known as a simple harmonic oscillator. One such set of movements is an Oscillation. In this chapter we explore a topic with huge implications for a lot of different subjects in physics. The motion of the block on a spring in SHM is defined by the position x(t) = Acos\(\omega\)t + \(\phi\)) with a velocity of v(t) = −A\(\omega\)sin(\(\omega\)t + \(\phi\)). The period T is the time it takes the object to complete one oscillation and return to the starting position. Simple harmonic motion is often modeled with the example of a mass on a spring, where the restoring force obey’s Hooke’s Law and is directly proportional to the displacement of an object from its equilibrium position.